How We Managed What Einstein Thought Was Impossible – And Used His Theory To Weigh A Star

MessageToEagle.com – If you are looking at a glass lens you will not see the lens itself, but rather notice objects behind it being displaced and distorted. Gravitation has a similar effect.

Any massive body warps the space and time around it, according to Albert Einstein’s theory of general relativity. As a result, light rays should take an apparent turn around the object rather than travelling in a straight line.

In 1919, during a solar eclipse, Arthur Eddington was able to show that this is true. He measured the apparent displacement of stars caused by the bending of their light around the sun, which acted as a “gravitational lens”. It was this result that made Einstein rise to instant fame. Now, a century later, we have for the first time measured this effect on a star other than our sun – something Einstein himself thought was impossible. Our results have been published in Science.

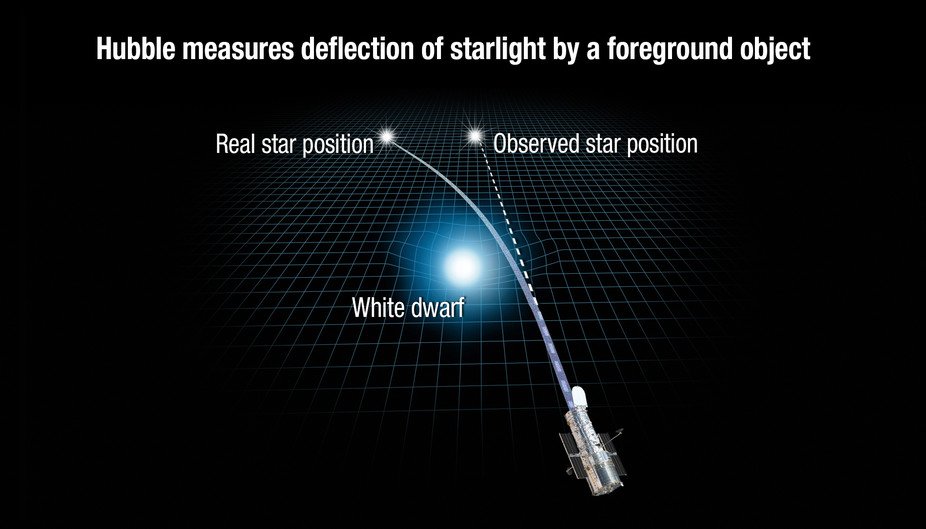
Using the Hubble Space Telescope repeatedly over the course of two years (from October 2013 to October 2015), we monitored the changing position of a background star at the moment it moved to be closely aligned with the nearby white dwarf star Stein 2051 B. White dwarfs are of peculiar interest because, along with neutron stars and black holes, they are faint or invisible remnants of stars at the end of their lives.

Perfect scale

The “gravitational lens” works like a weighing scale, with the light deflection of the background star being analogous to the movement of the needle on the scale. That’s because gravitational strength depends on mass – the bigger the mass, the bigger the effect of gravitational lensing. Consequently, after spending a further year and a half on careful analysis of the acquired data, we were able to directly obtain the mass of Stein 2051 B from the measured deflection of the background star. Stein 2051 B turned out to be 68% the mass of the sun.

While Eddington measured an already incredibly small angle of 1.7 arcseconds – roughly corresponding to the diameter of a human hair seen from 10 metres distance – the measured shift of the background star aligned with Stein 2051 B was 1,000 times smaller, up to 0.002 arcseconds. This reflects the fact that the space curvature is quite small.

In fact, the bending of light in curved space is quite similar to a ball rolling along the surface of Earth. While the Earth’s surface looks flat to us at first sight as we stand on it, the rolling ball follows its small curvature rather than moving strictly in a straight line. After rolling just about 6cm, its direction will have changed by 0.002 arcseconds.

See also:

Dr. Thomas Harvey – The Pathologist Who Stole Einstein’s Brain

New ‘Einstein Ring’ – A Rare Image Of A Distant Galaxy – Discovered

Einstein’s Brain Was Unlike Those Of Other People

Despite the huge success of Eddington’s observations of light bending by the sun, Einstein was sceptical about the prospects for observing this for other stars. In 1936, he concluded: “Of course, there is no hope of observing this phenomenon directly.” What he could not have predicted were the technological advances of the decades to come, such as the advent of fast computing engines and digital cameras.

The bending of light by stars is known as “gravitational microlensing”. Unlike the arc-like shapes of galaxies resulting from gravitational lensing (see image above), this weak phenomenon does not lead to observable image distortions. Crucially, it depends on a close alignment between background and foreground stars, which is quite rare. In principle, a foreground star creates two images of the background star, differing in luminosity. Their combined light can then lead to an apparent brightening of the background star as the intervening foreground star passes near the line of sight.

This effect, known as “photometric microlensing”, has been observed lots of times before. However, the measured positional shift of the star passing by Stein 2051 B marks the first ever observation of “astrometric microlensing”.

This latter effect holds the potential to shed new light on how stars evolve by surveying stellar remnants (white dwarfs, neutron stars and black holes) in our neighbourhood – along with brown dwarfs (“failed” stars not massive enough to sustain the nuclear fusion of hydrogen). These otherwise escape detection due to being faint or invisible, but gravitational lensing relies solely on their mass rather than their light.

By the end of its mission in 2019, ESA’s Gaia satellite will have found astrometric microlensing signatures that will provide reliable mass measurements for more than a thousand bodies, turning astrometric microlensing from a most curious effect into a useful astrophysical tool.

MessageToEagle.com

This article was originally published on The Conversation. Read the original article.